Previously, we looked at how to sample points randomly at a given density across an infinite plane.
It’s harder than it sounds, as I was looking for an algorithm that was not biased by the size/shape of the chunks used to calculate it.
Today let’s extend that to filling the infinite plane with random non-overlapping rectangles. As before, that means finding a deterministic chunked algorithm that we can prove is unaffected by the choice of chunking.
Continue readingI’ve added an article on handling infinite sized procedural generation to the Sylves documentation. It’s probably of interest to readers of this blog as the techniques are fairly general.
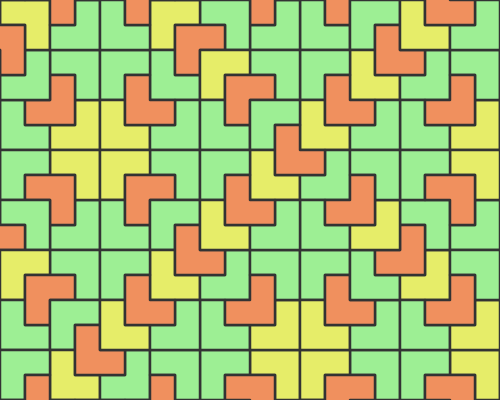
Everyone loves the Townscaper grid. It’s got a a nice organic look, while still being quadrilateral tiles, somewhat regular, and reasonably easy to implement. But it has some annoyances. I’ve finally managed to find my own design of grid that has a very similar look, but fixes these problems.
Continue reading
In procedural generation, the absolute simplest, most common technique is randomly picking an item from a list. More often than not, it is a weighted random choice, where each item is selected with a frequency proportional to its “weight”, a numerical value that can be tweaked by the designer.
def random_choice(items: list, weights: list[float]):
total_weight = sum(weights)
random_value = random.random() * total_weight
# Find the item that corresponds to the random number
for i, weight in enumerate(weights):
random_value -= weight
if random_value <= 0:
return items[i]I wanted to share a technique from the Machine Learning community that is simple enhancement to this routine that gives a lot of convenience over tweaking weights directly, called temperature.
Continue reading
Last week, I saw a talk on Vuntra City, a procedurally generated city with a fully explorable city. Developer Larissa Davidova explained that she settled on using Recursive Subdivision for the city blocks, as she wanted some level of organicness, while still only having to deal with rectangles. But she didn’t like having indefinitely long roads that cause implausible sightlines.
One way Vuntra City handles this is by subdividing a rectangle into 5 blocks, a pattern I called “whirl” in my previous article on recursive subdivision. You can see that it has no internal roads that stretch across the entire map.
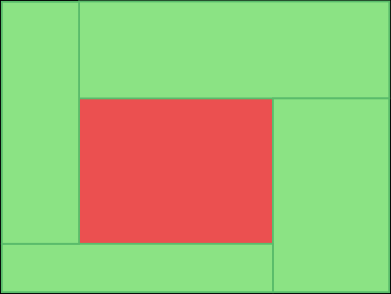
But Larissa’s talk got me thinking. The whirl pattern is interesting because it cannot be made from simple cuts. What other ways of subdividing a rectangle into smaller rectangles1, are out there?
Continue reading
Tracery bots were a fun, simple, way of making generative texts. They are basically an easy way to specify generative grammars via a simple JSON file format. There used to be a horde of fun little tracery bots on twitter until API changes shut them all down.
Nowadays, you can prompt a chatbot to get whatever you want. But that lacks the same charm, and it doesn’t give you the control you’d want for something unleashed on the internet. Let’s do something about that.
Continue reading
Recently I’ve been playing around more with gen AI techniques. I thought I’d try to generate a set of tiles that all connect together. It’s harder than it sounds – Stable Diffusion is hard to control, so there’s no easy way to get a set of images that are fully consistent with one another.
I’ve developed a technique for doing it that I’ll call Non-Manifold Diffusion as it involves doing diffusion over a set of patches that interlock to form a non-manifold surface.
Continue reading
I went to some lectures on the future of science in games recently, and the keynote speaker was Tommy Thompson, an well-known AI expert in the game dev space.
Of course, by AI, he didn’t mean the modern sort that dominates the news. His focus is AI for games, which is algorithmic and rarely involves any ML component. Still, he spoke about the challenges the industry faces regarding Image Generators, LLMs and so on. He specifically called LLMs “stochastic parrots”, which I found disappointing. Imho it’s an incredibly misleading model of what LLMs are capable of and is usually deployed to downplay their abilities and belittle them. But it’s a common view, particularly in creative industries.
So what is a better model? It’s clear that they are not that smart in most ways we consider important, but they do have some interesting capabilities. Here’s model I use that I feel give a better intuition for what they can and cannot do.
Continue reading
I’ve been working on adding aperiodic grids to Sylves.
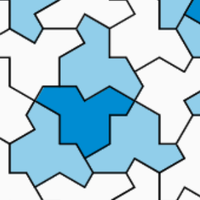
Aperiodic tilings are made tilings are made of a fixed set of tiles, rotated and translated to fully cover the plane.But they are not periodic – there’s no way to rotate/translate the whole grid onto itself.
This makes them almost hypnotic in their balance of regularity and chaos. A classic example is the penrose tiling.
One of my biggest gripes with the WaveFunctionCollapse procedural generation algorithm is that, despite the name, it doesn’t really have anything to do with quantum mechanics. I usually prefer the term Constraint Based Procedural Generation instead.
The name WaveFunctionCollapse is meant more as an analogy. As the algorithm progresses, it resolves a fuzzy, uncertain picture of the output into sharper detail, much as in quantum mechanics, the state of a system is also a range of possibilities, which resolves to something specific when “observed”.
But could we adapt WFC to the Quantum way of thinking, and ran it on actual Quantum Hardware? Well, that’s exactly what is discussed in this new paper Quantum WaveFunctionCollapse by Raoul Heese1 (Youtube summary). Does it work? Is it fast? Let’s find out.
Continue reading